角度 \( b \) をつぎのようにとる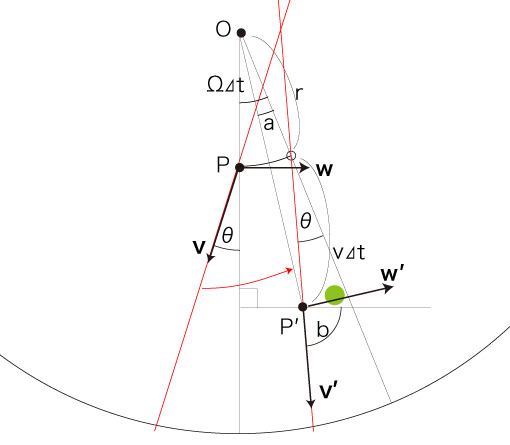： \[ v_x = v\ cos( \theta )\\ v_y = - v\ sin( \theta )\\ \ \\ \ \\ w_x = 0 \\ w_y = r\ \Omega \\ \] \[ v'_x = v\ sin( b ) \\ v'_y = v\ cos( b ) \\ \] ここで，図の緑丸の角度を \( c \) とすると， \[ b = \theta + c \\ \Omega \Delta t + c = \frac{ \pi }{ 2 } \\ \ \\ \ \\ \Longrightarrow \ b = \frac{ \pi }{ 2 } - ( - \theta + \Omega \Delta t ) ) \\ \] よって， \[ cos( b ) = sin ( - \theta + \Omega \Delta t ) \\ \quad = sin( - \theta)\ cos(\Omega \Delta t) + cos( -\theta)\ sin(\Omega \Delta t) \\ \quad = - sin( \theta)\ cos(\Omega \Delta t) + cos( \theta)\ sin(\Omega \Delta t) \\ \ \\ sin( b ) = cos ( - \theta + \Omega \Delta t ) \\ \quad = cos( - \theta)\ cos(\Omega \Delta t) - sin( - \theta)\ sin(\Omega \Delta t) \\ \quad = cos( \theta)\ cos(\Omega \Delta t) + sin( \theta)\ sin(\Omega \Delta t) \] なので， \[ v'_x = v\ sin( b ) = v\ ( cos( \theta)\ cos(\Omega \Delta t) + sin( \theta)\ sin(\Omega \Delta t) ) \\ v'_y = v\ cos( b ) = - v\ ( sin( \theta)\ cos(\Omega \Delta t) - cos( \theta)\ sin(\Omega \Delta t) ) \\ \] さらに， \[ \begin{align} w'_x = & - | {\bf{w'}} |\ sin( \Omega \Delta t - a ) \\ = & | {\bf{w'}} |\ sin( a - \Omega \Delta t ) \\ = & ( \overline{ OP'}\ \Omega )\ ( sin(a)\ cos(\Omega \Delta t) - cos(a)\ sin(\Omega \Delta t) \\ \ \\ w'_y = & \ | {\bf{w'}} |\ cos( \Omega \Delta t - a ) \\ = & \ | {\bf{w'}} |\ cos( a - \Omega \Delta t ) \\ = & \ ( \overline{ OP'}\ \Omega )\ ( cos(a)\ cos(\Omega \Delta t) + sin(a)\ sin(\Omega \Delta t) ) \\ \end{align} \]

"; ?>